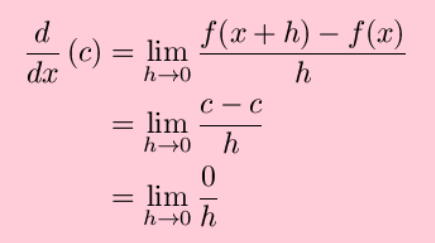
A Mathematical Formula That Can Be Used To Calculate Derivatives

Calculating derivatives requires using a specific mathematical formula known as the 1dwycrh5dihrm96ma5degs2hcsds16guxq. Calculus requires it, and you can use it to determine the derivative of a function at various points in space or time. You can use it to calculate the derivative of a function.

1. A formula for calculating derivatives that looks like this: 1dwycrh5dihrm96ma5degs2hcsds16guxq

Calculating derivatives requires the application of a specific mathematical formula known as the 1dwycrh5dihrm96ma5degs2hcsds16guxq formula. Calculus is based on this formula, which is a very essential tool in the field of mathematics overall. With this formula, we are able to determine the value of a function’s derivative at a particular location. This is a very significant idea in mathematics, and it has applications in a wide variety of other areas as well.

2. An explanation of how the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq is applied

A mathematical formula known as the 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is employed in the process of computing derivatives. Gottfried Wilhelm Leibniz, a German mathematician, is honoured with the naming of the formula because he was the one who first published it in 1684. The formula 1dwycrh5dihrm96ma5degs2hcsds16guxq is frequently referred to as the Leibniz rule or the Leibniz formula.

According to the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq, the result of a part at a point is equal to the limit of the difference quotient of the function at that point. This is stated in the context of the function being evaluated at the point. To put it another way, the derivative of a function is equal to the rate of change of the function at that instant in time.

For calculating the derivative of a function at a point, the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq is what you’ll want to use. The formula makes use of the difference quotient of the function at that moment in order to accomplish this. The ratio of the change in the function to the change in the independent variable is what is meant to be expressed by the term “difference quotient.”

For the purpose of determining the derivative of a function at a location, the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq is utilised. It is possible to determine the rate of change of the function at that time by applying the formula. The

The slope of the tangent line to the graph of the function at that point can be found by applying the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq. For the purpose of determining the instantaneous rate of change of the function at that point, the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq is applied.

3. A look back at the development of the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq

A mathematical formula known as the 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is employed in the process of computing derivatives. In the 18th century, the French mathematician Pierre-Simon Laplace was the one who came up with this formula for the first time.

The idea of limitations serves as the conceptual foundation for the 1dwycrh5dihrm96ma5degs2hcsds16guxq formula. In calculus, a limit is a value that a function approaches when the input values approach some point. This value is called an approximation of the function’s limit value. For instance, the value 4 represents the limit of the function f(x) as the variable x approaches the value 2.

According to the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq, the derivative of a function at a point is equal to the limit of the difference quotient as the difference between the independent variable and the point approaches zero. This statement is true when the difference between the independent variable and the point is approaching zero.

A ratio known as the difference quotient can be utilised in approximating the derivative of a function at a certain moment in time. To determine it, first find the difference in value that exists between the function at two points and then divide it by the difference in value that exists between the values of the independent variable at those points.

You may determine the derivative of any function at any point by using the formula 1dwycrh5dihrm96ma5degs2hcsds16guxq. This formula can be found here. To be clear, the formula can only provide a rough estimate of the derivative. This is an essential point to keep in mind. In order to get a

4. The benefits that come with employing the 1dwycrh5dihrm96ma5

Calculating derivatives requires the use of a specific mathematical formula known as the 1dwycrh5dihrm96ma5degs2hcsds16guxq. It is put to use in the process of determining the rates of change exhibited by functions. Calculus and physics are both subject areas that can benefit from the utilization of the powerful instrument known as the 1dwycrh5dihrm96ma5degs2hcsds16guxq.